Where
k0 is known as the radius of gyration of the rigid body with respect
to an axis passing through 0.
The moment of
inertia of the rigid body with respect to the center of gravity will be given
by:
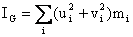
We can write
I0 as:
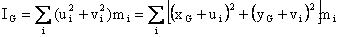
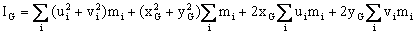
Since
ui and vi are the coordinates of the particle with respect
to the center of gravity Suimi
= Svimi
= 0. Therefore:
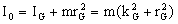
Where
kG is the radius of qyration about the center of gravity and
rG is the magnitude of the position vector of the centar of gravity
from 0. We thus have a fundamental theorem:
6.4.2. Parallel
Axis Theorem:
The
moment of inertia of a body about any axis is equal to the moment of inertia
about a parallel axis passing through the center of gravity plus the product of
the mass of the rigid body and the square of the distance between the two
axes.
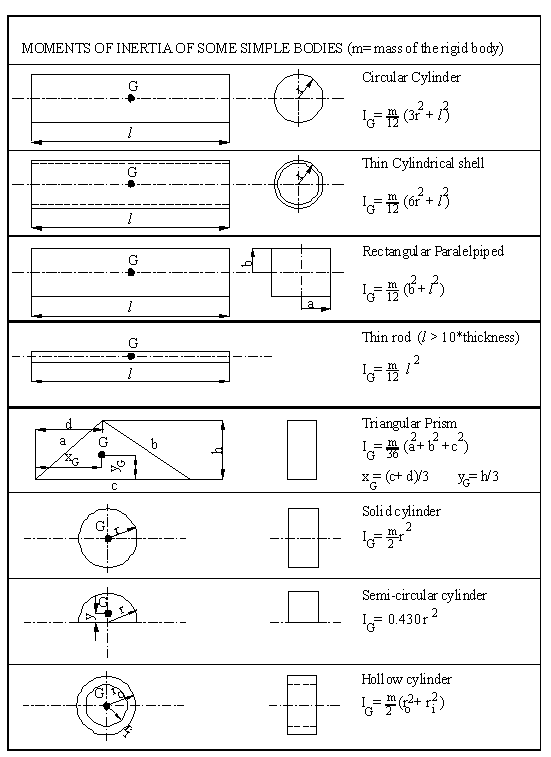
In the following
table moments of inertia of some simple bodies are shown: For bodies of a
complex shape the moments of inertia can be computed by separating the rigid
body into some simple shapes and applying parallel axis theorem. Usually, if a
drawing package with a solid modelling is used, the mass and the moments of
inertia of the parts can be determined automatically by these packages. In
general, the actual manufactured pieces may differ from the drawings in terms of
the mass and the moment of inertia. In cases where the moment of inertia of an
existing part is required experimental methods are used to determine these
quantities.
6.4.3. Newton's
Second Law of Motion for a Rigid Body
According to
Newton's second law the rate of change of momentum of a particle is proportional
to the resultant external force acting on the particle. For a particle in the
rigid body of constant mass mi , Newton's second law
becomes:
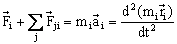 (1)
(1)
Where
Fi is the external force acting on particle i and
Fji is the force acting on particle i due to particle j.
Fji is commonly known as the internal force. If we consider all the
particles within the rigid body:
 (2)
(2)
Noting
that:
 = the sum of all
the external forces acting on the rigid body.
= the sum of all
the external forces acting on the rigid body.
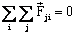 since Fij + Fji = 0 due to Newton's
third law.
since Fij + Fji = 0 due to Newton's
third law.
and
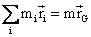 .
.
We thus
have Newton's Second Law of motion for
Linear momentum for a rigid
body, which is:
 (I)
(I)
From this
equation one can see that the internal force has no effect on the motion of the
rigid body, the center of gravity behaves as if the whole mass were concentrated
and the resultant force SF
were acting upon
this point. The term mvG is known as the linear momentum of
the rigid body.
Now if we take
the moment of the forces in equation (1) with respect to point 0, we
obtain:
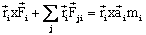 (3)
(3)
The acceleration
of point i, ai, can be written in terms of the acceleration of
point 0 and relative accelerations as:
ai =
a0 + ai/0 = a0 +
ai/0 n+
ai/0t
Where
ai/0t and ai/0 n are
the relative tangential and normal accelerations of point i with respect to
point 0. In vector notation these terms are given by:
ai/0
t= 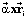
ai/0
n= -w2ri
Where
a and
w are the angular
acceleration and velocity of the rigid body respectively.
Substituting the
above relations into equation (3) and summing up for all the particles within
the rigid body results in:
 (4)
(4)
Noting
that:
-
 = sum of the
moment acting along an axis passing through O (perpendicular to the
plane).
= sum of the
moment acting along an axis passing through O (perpendicular to the
plane).
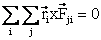 (since
Fij = -Fji and these forces have
same line of action. The summation will be composed of terms
(since
Fij = -Fji and these forces have
same line of action. The summation will be composed of terms 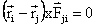
The terms
on the right hand side of equation (4) can be written as:
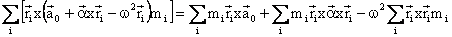 (5)
(5)
Now :

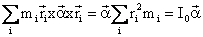
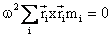
Hence, equation
(4) simplifies into:
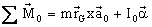 (6)
(6)
In general
 . The first term
on the right-hand side of equation (v) will vanish if
a0=0 or rG=0 or if a0 and
rG are parallel. The acceleration of point 0 is zero if
point 0 is the acceleration pole or if the rigid body is in a rotation about
point 0.
. The first term
on the right-hand side of equation (v) will vanish if
a0=0 or rG=0 or if a0 and
rG are parallel. The acceleration of point 0 is zero if
point 0 is the acceleration pole or if the rigid body is in a rotation about
point 0.
rG = 0 means that
point 0 coincides with the center of gravity G and a0
will be parallel to rG only in very special cases.
However in any general case the center of gravity can be made coincident with
the center of the reference axis. We thus have Newton's Second Law of Motion for Angular
Momentum of a rigid body, which is:
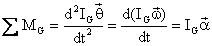 (II)
(II)
Note that both
the moment of external forces and the moment of inertia of the rigid body must
be with respect to an axis passing through the center of gravity and
perpendicular to the plane of motion. The term IGw is known as the
angular momentum of the rigid body with respect to center of
gravity.
6.4.4. D'Alambert's
Principle
Equations I and
II can be written in the following form:
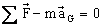 (I)
(I)
and
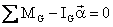 (II)
(II)
Now the term 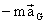 has the magnitude of a force. Equation I is a vector
equation which states that the vector sum of all the external forces plus the
fictitious (nonexistant) force of magnitude and direction
has the magnitude of a force. Equation I is a vector
equation which states that the vector sum of all the external forces plus the
fictitious (nonexistant) force of magnitude and direction 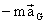 are
zero. The fictitious force
are
zero. The fictitious force 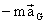 is known as the
inertia force which will be
denoted by Fi:
is known as the
inertia force which will be
denoted by Fi:
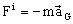
Fi has the same
line of action of aG but is in opposite direction.
Similarly, the
term 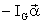 has the magnitude of a moment and equation II is a vector
equation which states that the vector sum of all the external moments about the
center of gravity plus a fictitious moment of magnitude and direction
has the magnitude of a moment and equation II is a vector
equation which states that the vector sum of all the external moments about the
center of gravity plus a fictitious moment of magnitude and direction 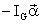 are
zero. This fictitious moment is known as the inertia torque and it will be denoted by
Ti :
are
zero. This fictitious moment is known as the inertia torque and it will be denoted by
Ti :

Ti is in
opposite sense of the angular acceleration a. Using inertia
force and inertia torque Newton's Second Law of Motion for a rigid body results
with the equations:
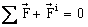
and
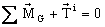
We can as well
treat the inertia terms as if they were another external force or moment acting
on the rigid body, in which case:
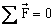
and
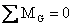
Where the
summation includes both the external and inertia forces and moments. This
concept is known as D'Alambert's principle which can be stated as
follows:
D'Alambert's
Principle
In a body moving with a known angular acceleration and a linear acceleration of
the center of gravity, the vector sum of all the external forces and inertia
forces and the vector sum of all the external moments and inertia torque are
both separately equal to zero.
D'Alambert's
principle is very useful in the dynamic force analysis of machinery. In great
many problems we know the acceleration characteristics of the members in the
machine structure and we can determine the inertia forces and torques. These
inertia forces and torques can be treated as if they are external forces and the
procedure of static force analysis can be carried out for this dynamic case.
However one must never think the inertia forces and moments as real forces. They
are fictitious forces and they never exist. Acceleration is a result of the
external forces.
In graphical solutions it is convenient to replace the inertia force and torque
by an equivalent resultant inertia force.
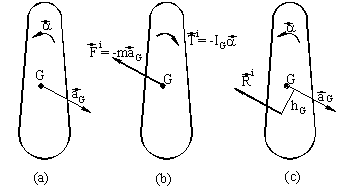
Consider a rigid body with aG as the acceleration of its
center of gravity and a as its angular
acceleration (Figure.a). The inertia force and torque will be as shown in Figure
b. The inertia force and torque can be combined into a single resultant,
Ri (Figure c) , if:
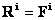
and
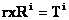
where r
is a position vector from the center of gravity to a point on the line of
action of Ri. In such a case the resultant
Ri has the magnitude and direction of the inertia force
Fi and is displaced from the center of gravity by a
perpendicular distance hG such that:

This fictitious
force Ri will then replace the effect of the inertia force and
torque

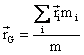
![]()
![]()